With the release of Mountains® 8 comes the possibility to load and visualize a new kind of data, known as “Shells” or “Freeform surfaces”.
Christophe Mignot, Digital Surf CEO takes a closer look at this new data type and explains how it’s different from standard surface data.
To begin with, what is a “standard” surface?
Nowadays, most surface texture and form analysis is based on what we call “surface topography”. The type of data which corresponds to this is simply referred to as a “surface” in Mountains® software.

A surface is stored as data of type z=f(x,y). The expression z=f(x,y) just means that the height depends (“is a function of”) the lateral position (x,y).
Rule 1 : data is regularly spaced over X and Y directions.
Hence there is no need to store X and Y values. In fact, one could say that a surface is a kind of Excel table where the height is the content of the cells, rows and columns representing the X and Y coordinates. (Spacing between two successive rows and between two successive columns is constant and each is only stored once in the object header).
Rule 2 : There can only be ONE height for any given X,Y position.
This is fundamental. An “Excel cell” can be empty (we call it a “non-measured” point) but in no case can there be two or more values in the same cell.
Let’s see the consequences by taking a concrete surface texture example. Suppose for instance, you want to study the average heights of the bumps of a pickle.

A standard “surface” will only let us characterize an extracted, relatively flat, portion of the pickle’s external surface. As there can only be one Z height value (or Excel cell) for a given X,Y horizontal position, this type of data cannot manage both the top and bottom side of the pickle. So using only standard “surface” data, it is impossible to calculate the average roughness of the common pickle!
This is also true for:
- Vertical slopes. As there is only one Z value for any given X,Y horizontal position, a vertical surface cannot be encoded. In order to be measured and studied, it must be rotated.
- Overhangs. Same problem. If there is an overhang, there is more than one Z value for some X,Y positions.
- Deeply porous surfaces. These contain many small overhangs. For instance sponges or agglomerated grains such as objects produced using additive manufacturing are not “standard surfaces”.
- Internal cavities such as those visible using X-Ray tomography.
Using surfaces has of course brought great service to many over the years. Digital Surf has pioneered surface analysis since 1989, offering topography analysis at a moment when the industry was only using profiles, i.e. height along a single line segment z=f(x), (equivalent of a single row in Excel).
“Surfaces” are sufficient for many applications, and remain a core part of Mountains® software. However, it is time to go a step further. Among other new types of studiable, Mountains® 8 introduces freeform surfaces or “shells”.
So what is a “Shell” and why is it different?
In order to be able to describe the whole surface around (or even inside) objects, mathematicians usually describe the surface as a long list of small triangles (known as «facets»). Each triangle is defined by three summits. Contrary to standard surfaces, there is no grid and no natural order. Two facets are neighbors when they share two summits, end of story.
Mountains® 8 can now manage these “freeform surfaces”. This name is meaningful but quite long and so to fit nicely in Mountains®, we found a shorter one. We simply call our freeform surfaces, “shells”. Check out this image of a peanut shell and you will understand why.

We chose the peanut shell as the new icon for the “shell” studiable in Mountains®. It is reminiscent of the existing icon for surfaces, but clearly express the new ability to go all the way around the object.
What can I do with a shell data in Mountains® 8?
Implementation of the new “Shell” data type implies the “basic” services (as for all studiables):
- Load main types of file formats for freeform surfaces (.STL, .OBJ, .PLY)
- View data
- 3D-Print: Mountains® 7 is already able to 3D-print standard surfaces; the feature is now extended to shells. The main application for this is producing accurate centimetric/inch-scale objects that can be manipulated from data at the sub-millimeter scale. This is of obvious educational and scientific interest.
Since Mountains® software is already expert in standard surface analysis, we also decided to develop a way of obtaining surfaces and profiles from shells, so that users could benefit from already existing tools.
A small zone of the surface of a shell can be extracted and analyzed the same way as standard topography. A cross section can be extracted from a shell and analyzed just like a standard contour (parametric profile allowing loops).
What are the main applications of shell data?
In Mountains® 8 users can open data produced by profilers with multiple scanning axes which produce complex shapes (gears etc.)
They can input (external and internal) shapes and textures of objects produced by additive manufacturing and scanned using X-ray tomography.
It is also possible to view and manipulate such objects as you please, and extract any external or internal face or cross-section at any angle. The extracted data can then be analyzed using Mountains® numerous surface and contour analysis tools for dimensional control and surface texture analysis.
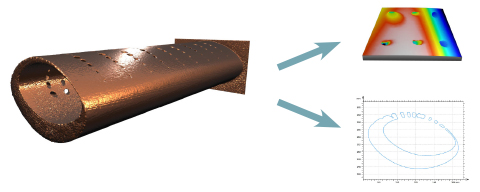
Shell data courtesy of University of Huddersfield, UK
What’s next for shells?
Ultimately, our goal is to be able to offer surface texture parameters for shells. Remember the pickle? This would correspond to an Rq value calculated all around the pickle that would assess the global average roughness of the pickle surface (as opposed to extracting a surface first in order to calculate standard surface roughness parameters).
However, today there is no standard for such parameters. Along with other members of the scientific community, we are still at work on the subject. So at this time there are no “Parameters for shells” in Mountains®… but stay tuned!
Author : Christophe Mignot
Resources :
- Scott, P. J., & Jiang, X. (2014). Freeform surface characterisation: Theory and practice. Journal of Physics: Conference Series, 483(1), [012005]. https://doi.org/10.1088/1742-6596/483/1/012005
- Leach, R., De Groot, P. & Haitjema, H. (2018). Challenges of high slopes and complex features in the metrology of structured and freeform surfaces. Conference paper (Euspen Structured & Freeform Surfaces).
- Surface Metrology Guide: digitalsurf.com/guide